Find the parametric equations for the line through the point

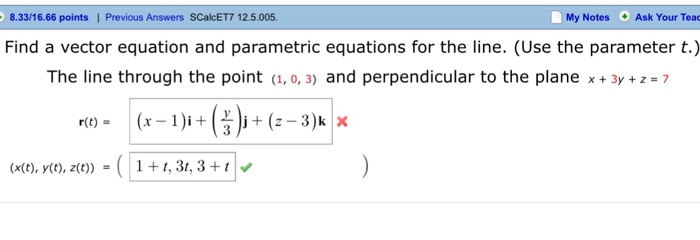
X 2ty 1 tz t Symmetric equations. So Im just writing all the components and writing them out in one single vector.

Where r 0 r_0 r 0 is a point on the line and v v v is a parallel vector.

Find the parametric equations for the line through the point. The basic data we need in order to specify a line are a point on the line and a vector parallel to the line. That is we need a point and a direction. P 0 point P x y z v direction Example 1.

Write parametric equations for a line through the point P 0 1 2 3 and parallel to the vector v 1 3 5. If P xyz is on the line then the vector P. The parametric equations of the line passing through two non-coinciding points x1y1z1 x 1 y 1 z 1 and x2y2z2 x 2 y 2 z 2 are given by.

X x1dxt y y1dyt z z1dyt x x 1. A parameterization of a line has the form rt P t D where P is a vector touching the line and D is a direction vector for the line. Given two points on the line P and Q the equation rt P t Q is not the correct parameterization.

Therefore the parametric equations of a line passing through two points P 1 x 1 y 1 and P 2 x 2 y 2 x x 1 x 2 - x 1 t y y 1 y 2 - y 1 t. So in this problem we want to find the Parametric equation for line. Its through this 03 minus 21 Its called that p and parallel to the line given by this equation.

So maybe another waiter right. This line thats given to us in vector form would be like this. So Im just writing all the components and writing them out in one single vector.

Find parametric equations of the line passing through point P-213 that is perpendicular to the plane of equation 2 x-3 yz7 Answer We show that the parametric equation of the required line. A vector equation for the line is rt x0 y0 z0 t a b c r t x 0 y 0 z 0 t a b c and the parametric equations for the line are x x0at y y0 bt z z0ct x x 0 a t y y. Find the vector and parametric equations of the line segment defined by its endpoints.

P 1 2 1 P 12-1 P 1 2 1 Q 1 0 3 Q 103 Q 1 0 3 To find the vector equation of the line segment well convert its endpoints to their vector equivalents. Find the parametric eq of the line through points 13 -4 and 321. Constructing a vector we get 3-1 2-3 14 2 -1 5 point on line Let r represent a point on the line l.

Then r 2 -1 5 t13-4 or r 2 -1 5 t321 Which can I choose as my parallel vector in this case. The vector equation of line is 8i-7j Ekt tl it yjZk tityj zk 18 t j -7 5t k 6-26 parametric equation of the line is given by Xt 8t yt -755 2t 6- 2 The equation of the plane through the point 178. And with normal vector 93 j-k.

We have The equation of plane passing through the point is GarryZ and with normal vector aitojt ck given by ax-X by-Y 2-20. Rt Parametric form parameter t and passing through P whent 0. 1 point Find the vector and parametric equations for the line through the point P -11-4 and parallel to the vector 0 -3 -2.

Rt Parametric form parameter t and passing through P when t 0. Z t y yt z zt. Where r 0 r_0 r 0 is a point on the line and v v v is a parallel vector.

The parametric equations of a line are given by. X a xa x a. Y b yb y b.

Z c zc z c. Where a a a b b b and c c c are the coefficients from the vector equation r a i b j c k. Find the vector and parametric equations for the line through the point P523 and the point Q278.

Your answer Email me at this address if my answer is selected or commented on. Email me if my answer is selected or commented on. 83 Vector Parametric and Symmetric Equations of a Line in R3 2010 Iulia Teodoru Gugoiu - Page 1 of 2 83 Vector Parametric and Symmetric Equations of a Line in R3 A Vector Equation The vector equation of the line is.

R r0 tu tR r r r where. Ö r OP r is the. A point on the line.

210 A direction vector. Ij jk h110ih 011i i j k 1 1 0 0 1 1 i jk Vector equation. 11i h2t1 tti Parametric equations.

X 2ty 1 tz t Symmetric equations. X 2 1 y 1 1 z 1 or x 2 1 y z 12515aFind symmetric equations for the line that passes through the point 1. 56 and is parallel to the vector h 12.

X 1 1 y5 2 z 6 3 bFind. Find a vector equation and parametric equations for the line. Use the parameter t The line through the point 8 7 6and parallel to the vector 15 -23 rt xtytzt 2.

Find an equation of the plane through the point 1 7 8 and with normal vector 9i j k 3. Standard line equation is ymxn where m is the slope n the y axis intersection. So for a line defined by two points the eq is y-y0 y1-y0 x1-x0 x-x0 for parametric equation is defined by a point A and the director vector applied on A.

Being perpendicular to a triangle is the same as being perpendicular to the plane containing the triangle. To determine the equation of a line in 3-D you need a point on the line which you have and a direction vector for the line. Call your triangle ABC.

Use the coordinates of points A. Find a vector equation and parametric equations for the line. The line through the point 2 24 35 and parallel to the vector 3i 2j - k.